Absolute Value Function Vertex Form

FIND THE VERTEX OF ABSOLUTE VALUE EQUATIONS

In general, the graph of the absolute value office

f (x) = a| x - h| + k

is a shape "V" with vertex (h, k).

To graph the absolute value function, we should exist aware of the following terms.

Horizontal Shift :

Let us consider ii different functions,

y = |x| and y = |x-ane|

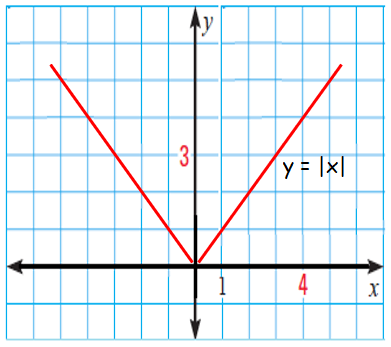
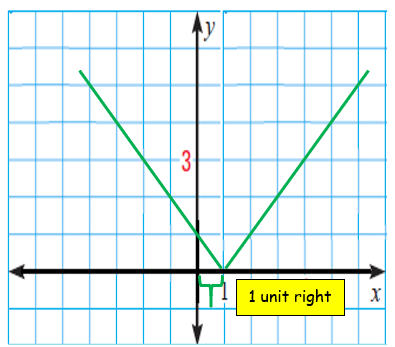
Vertex of the absolute value function y = |x| is (0, 0).

Vertex of the absolute value function y = |x-1| is (1, 0).

Past comparing the above two graphs, the second graph is shifted 1 unit of measurement to the right. Since the value h is > 0, we have to move the graph h units to the right side.

Conclusion :

If h > 0, motion the graph h units to the right.

If h < 0, movement the graph h units to the left.

Vertical Shift :

Allow us consider two different functions,

y = |x| and y = |ten| + one

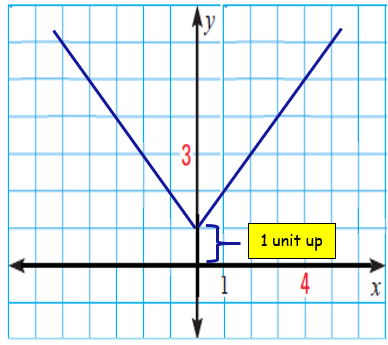
Vertex of the accented value function y = |x| is (0, 0).

Vertex of the absolute value role y = |10|+1 is (0, 1).

Instead of k, we accept +1. So, we have to motility the graph i unit up.

If the value of k is -ane. Nosotros have to move the graph 1 unit downwards.

Conclusion :

If 1000 > 0, motion the graph k units up.

If m < 0, move the graph 1000 units down.

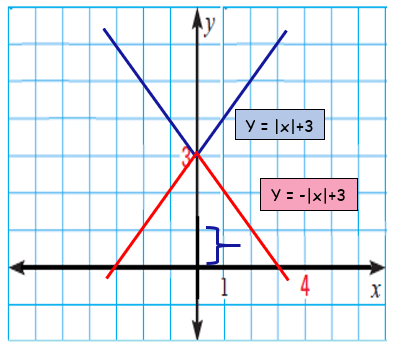
Stretch and Compression :

Let united states of america consider two different functions,

y = |x| + 3 and y = -|ten|+3

Graph the post-obit absolute value function.

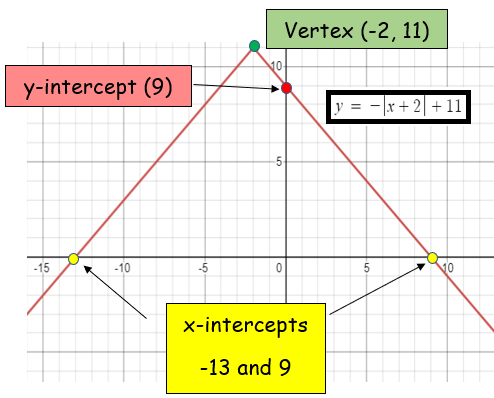
Instance 1 :

y = -|x + two| + 11

Solution :

To graph, let us find the following.

By comparing the given absolute value function with

y = |x - h| + k

Vertex (h, k) :

(-two, 11)

Horizontal Translation :

h = -2

Movement the graph two units to the left.

Vertical Translation :

g = xi

Move the graph 11 units up.

Stretches and Compressions :

a = -1

x-intercept :

Put y = 0.

0 = -|x + 2| + 11

-11 = -|x + ii|

|x + 2| = 11

(x + 2) = 11 and (x + 2) = -11

ten = ix and 10 = -13

y-intercept :

Put ten = 0.

y = -|0 + 2| + 11

y = -2 + 11

y = nine

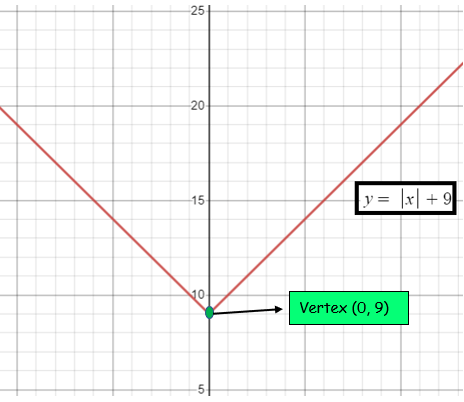
Example 2 :

y = |x| + 9

Solution :

To graph, allow usa discover the following.

Past comparing the given absolute value office with

y = |x - h| + thou

Vertex (h, chiliad) :

(0, ix)

Horizontal Translation :

h = 0

And then, no horizontal shift.

Vertical Translation :

thousand = 9

Move the graph 9 units up.

Stretches and Compressions :

a = ane

ten-intercept :

Put y = 0.

0 = |x| + 9

-9 = |10|

This will not happen, for whatever value of ten we will non become the answer -9. So, it has no ten-intercepts.

y-intercept :

Put x = 0.

y = |0| + 9

y = 9

Kindly mail your feedback tov4formath@gmail.com

We always appreciate your feedback.

© All rights reserved. onlinemath4all.com

Absolute Value Function Vertex Form,

Source: https://www.onlinemath4all.com/find-the-vertex-of-absolute-value-equations.html

Posted by: ingramshament.blogspot.com

Share this post